Spring 1997

PHYS 198 ASSIGNMENTS

Homework Assignment (1/15/97):

Problem 1 -- Lenses
A 2 inch high data image is to be transported through a 1/4 inch diameter fiber optic imaging cable to a CCD camera with a 1/2 inch high sensor. If the gap between the original image and the input end of the cable is 24 inches, while the gap between the output end of the cable and the CCD sensor is 12 inches, what are the lensing requirements?

Problem 2 -- Interference Fringes
If monochromatic light of 550 nm exits in phase from two very thin slits separated by 0.5 mm, how far apart (in mm) will the +/- 3rd order fringes be on a screen 1 m away?

Problem 3 -- Diffraction Envelope
If the slits in the previous problem are 0.1 mm in width, how far from the center (in mm) will the first missing interference fringe on either side be? What is the order of this missing fringe? (A fringe will be missing if a diffraction fringe minimum falls on top of an interference fringe maximum.)

Solutions to these problems will be found at Solutions (1-3).

Homework Assignment (1/27/97):

Problem 4 -- Polarization
The electric field vector of an incident linearly polarized light wave makes an angle of +30 degrees with the horizontal fast axis of a quarter-wave plate. Describe, in detail, the state of polarization of the emergent wave.
Problem 5 -- Fizeau Fringes
Fringes are observed when a parallel beam of light of wavelength 500 nm is incident perpendicularly onto a wedge-shaped film with an index of refraction of 1.5. What is the angle of the wedge if the fringe separation is 0.333 cm?
Problem 6 -- Grating Orders
Light having a frequency of 4.0 x 10¹⁴ Hz is incident on a grating formed with 10,000 lines per centimeter. What is the highest-order spectrum that can be seen with this device? Explain.
Problem 7 -- Diffraction from a square aperture.
Imagine that you are looking through a piece of square woven cloth at a point source (l_o = 600 nm) 20 m away. If you see a square arrangement of bright spots located about the point source, each separated by an apparent nearest-neighbor distance of 12 cm, how close together are the strands of cloth?

Solutions to these problems will be found at Solutions (4-7).

Reading Assignment:

Chapter 2. Sections 2.7 - 2.11. Wavefront interference and diffraction.
Chapter 3. Sections 3.1 - 3.3. Classical Interferometry. Newton's rings, Young's fringes, Michelson's interferometer.
Chapter 4. Section 4.1 - 4.5. Photoelasticity and birefringence.

Lab Assignment 1 (1/30/97):

Images from the diffraction and interference experiments done in the Lab on January 22, along with some analysis questions, are available by going to Diffraction Data Images.

Solutions to these questions will be found at Lab Set 1 Solutions.

Lab Assignment 2 (2/9/97):

Images from the photoelastic measurements performed in the Lab on February 5, along with some questions about getting numerical values from the images are available by going to Photoelastic Data Images.

Reading Assignment (2/14/97):

Chapter 7: Theory of Geometrical Moire
Chapter 8: Measuring strain by Moire

Lab Assignment 3 (3/10/97):

Images from the shadow and projection moire demonstration performed in the Lab on February 26, along with some questions and requested measurements are available by going to Moire Lab Images.

Reading Assignment (3/10/97):

Chapter 9, Section 9.1 : Shadow Moire.
Chapter 9, Section 9.3 : Projection Moire.
Chapter 10 : Diffraction and Fourier Optics. (Don't spend too much time on the math derivations.)

Homework Assignment - Fourier Transform Properties (3/17/97)

All of the Transform properties referred to in the problems are defined in the Lecture for March 17, 1997.
X(n) and Y(n) are the DFTs of x(k) and y(k), respectively.

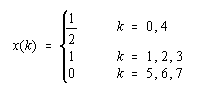
Hwset3eqn1

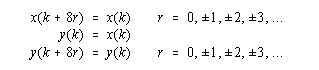
Hwset3eqn2

Problem 8. Compute X(n) + Y(n); add these results to get Z = X + Y.
Also calculate z(k) = x(k) + y(k). Do your results show the linearity property?
Problem 9. Demonstrate the symmetry property for x(k).
Problem 10. Compute the discrete Fourier transform of x(k - 3).
Compare your results with those obtained by using the time-shifting property of DFTs.

Solutions to these problems will be found at Solutions (8-10).

Reading Assignment (3/26/97) :

Chapter 16 : Holographic Interferometry Theory

Holography
Holographic Interferometry
Double Exposure
Real-Time
Vibrational
Classic Difficulties
Sensitivity Vector
Localization of Fringes

Homework Assignment - Frequency Shift Property and Display FFTs (3/26/97) :

Problem 11. It was mentioned in class that the 2-dimensional FFT of an image was generally transformed so as to put the (0,0) point of the x and y frequencies in the center of the FFT display. Limiting the discussion to one dimension, what would you do do a data set of N = 16 samples to center the zero frequency of its FFT on a display? (Hint: Start with the frequency shift theorem. You should end up with a general formula, not a calculation for 16 samples.)

Solution to this problem will be found at Solution 11.

Reading Assignment (4/14/96) :

Chapter 17: Holographic Interferometry Methods.

Chapter 18: Introduction to Speckle; what is speckle, objective vs subjective speckle, determinants of speckle size.

Homework Assignment - Holointerferometry Resolution Requirements and the Analysis of In-Plane Motion. (4/14/97) :

Problem 12. It has been frequently mentioned that holography requires a much higher resolution detector than ordinary photography. Assuming that a He-Ne laser beam (l = 0.633 mm) is split into two parts and then the two beams are brought together on a photographic plate with each beam at an angle f to the normal to the photographic plate, what is the spatial frequency of the grating that will be created on the plate in terms of f? Specifically, what will be the frequency of the grating for f = 5 degrees and f = 60 degrees? (Hint: Review section 2.9 of class textbook.)

Problem 13. The problem of the centrally-loaded disk with a fixed boundary was discussed at length in class, and is written up in the notes for the Lecture of April 9, 1997. Using the figures and data given in that Lecture, work out the maximum extent of the deformation undergone by the disk. Be sure to account for the magnitude of the sensitivity vector g.

Solutions to these problems will be found at Solutions (12-13).

Reading Assignment (4/24/97)

Chapter19, Sections 1-6 : Speckle Photography.

Chapter 20 : Speckle Correlation Interferometry.

Homework Assignment - Example of Speckle Photography Measurement (4/24/97) :

Problem 14. Answer the questions for the example given in the lecture notes for April 23, 1997.

Solution to this problem will be found at Solution 14.

Reading Assignment - ESPI, Phase Stepping, and Phase Unwrapping (4/29/97) :

Chapter 21, Sections 21.1-21.4, 21.9-21.10 : ESPI patterns, system resolution, advantages and limitations of ESPI.

Chapter 22, Sections 22.1-22.4, 22.6 : Phase shifting, especially 4-step, phase unwrapping.

Email questions or comments: matthysd@vms.csd.mu.edu

Last Modified on May 03, 1997

Visitors to this page :